Systematics of inhibition and nonessential activation

The model considered in the systematization of kinetic modifier mechanisms presented here is the general modifier mechanism published in 1953 by Jean Botts and her husband Manuel Francisco Morales [1] (Scheme 1). The detailed description of the underlying theories can be found elsewhere [2, Chapters 2 and 3], where also the reasons for choosing the adjectives specific in place of competitive, and catalytic in place of uncompetitive are explained. Balanced  applies to mechanisms in which the specific and the catalytic components are equally represented.

In the notation of the general modifier mechanism shown in Scheme 1 (left) the specific and the catalytic components of the reaction are highlighted with colors. K_Sp and K_Ca represent the dissociation constants of the specific and of the catalytic branch, respectively. The notation on the right of the scheme is more practical for writing rate equations and the dissociation constants K_X and αK_X refer to both inhibitors and activators. α is the reciprocal allosteric coupling constant, and β is the factor by which the modifier affects the catalytic constant k₂.

The taxonomy of enzyme-modifier mechanisms is based on the two characters α and β of the general modifier mechanism, where α is the dominant character. There are 17 basic mechanisms by which enzymes interact with inhibitors and nonessential activators. In the taxonomic tree of modifier mechanisms (Fig. 1) these are treated as species and ranking starts at the root on the top of the dendrogram as unmodified, active enzyme.

In Fig. 1, linear specific inhibition (LSpI, the same as competitive inhibition), and linear catalytic inhibition (LCaI, the same as uncompetitive inhibition) are considered primordial species without progeny, while linear mixed inhibition (LMxI, yellow-boxed) is the ancestor of the remaining fifteen mechanisms.

• The lineage with a green background has a specific or a predominantly specific character (1 < α < ∞)
• The lineage with an orange background has a catalytic or a predominantly catalytic character (0 < α < 1)
• In the lineage with a blue background the specific and the catalytic characters are equally represented (balanced, α = 1)

Within the three lineages, the secondary character β and its relationships with the primary character α establish when inhibition or activation occurs.

Proceeding from the root towards the bottom through levels 1-3, the mechanisms become more sophisticated in fine-tuning enzyme activity by either inhibition, activation or both in the same context. Therefore, the dendrogram in Fig. 1 represents the evolution from an ancestor to descendants, but just metaphorically, without phylogenetic pretenses, since the tree is based exclusively on phenetic, experimentally observable present-day characters.

With appropriate values of α and β, the rate equation of the general modifier mechanism (eq. 1) describes all mechanisms in Fig. 1:

 (1)    

In (1) the symbols in square brackets represent the concentrations of total enzyme [E]_t, free substrate [S] and free modifier [X], and K_m is the Michaelis constant. Equation (1) is valid under the equilibrium assumption, whose terms of validity have been discussed [2, Chapter 2]. Even if the equilibrium or the quasi-equilibrium assumptions are not valid, the expected deviations from the complete model based on the steady-state rate equation are masked by experimental errors, with the exception of extreme cases.

---

Definition of linear and hyperbolic enzyme modification

The definitions are based on the shape of the plots of the reciprocal catalytic constant (1/k_cat) and of the reciprocal specificity constant (K_m/k_cat) against [X], the modifier concentration. Both plots can be linear or hyperbolic, or at least one is either linear or hyperbolic when the other parameter is independent of [X].

• Inhibition is said to be linear  when at least one of the 1/k_cat or K_m/k_cat  plots against [X] is a straight line
• Inhibition and nonessential activation are hyperbolic when at least one of the 1/k_cat or K_m/k_cat  plots against [X] is a hyperbola (nonessential activation is in any case always hyperbolic)

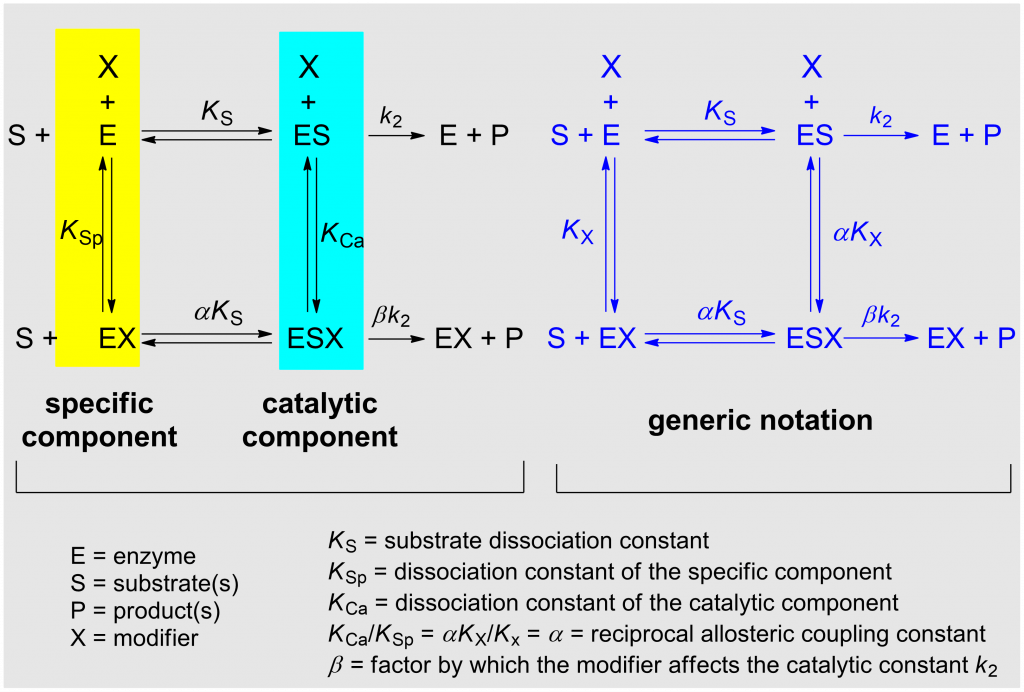
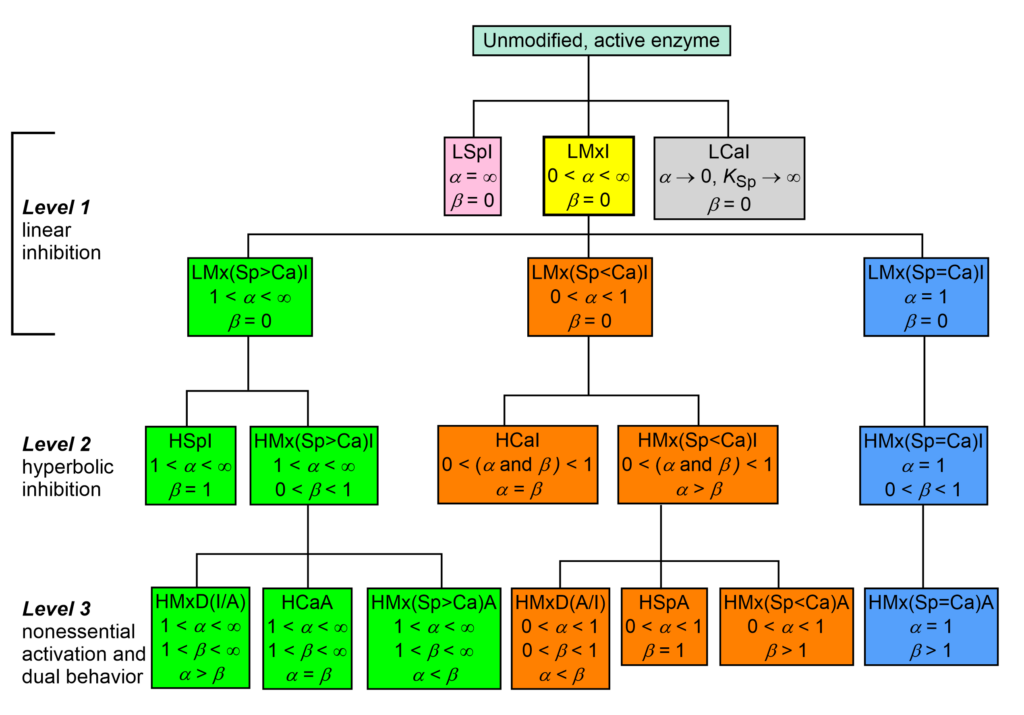
Fig. 1. Taxonomy of enzyme-modifier interactions. Zoom/Download

The acronyms in Fig. 1 are composed of the following elements:
A = activation, Ca = catalytic
D = dual, inhibition or activation, depending on substrate concentration
H = hyperbolic, I = inhibition, L = linear, Mx = mixed, Sp = specific
(Sp>Ca) = predominantly specific, (Sp<Ca) = predominantly catalytic
(Sp=Ca) = balanced, i.e. the specific and catalytic components are equally represented

ACRONYM	NAME
LSpI	Linear specific inhibition
LCaI	Linear catalytic inhibition
LMx(Sp>Ca)I	Linear mixed, predominantly specific inhibition
LMx(Sp<Ca)I	Linear mixed, predominantly catalytic inhibition
LMx(Sp=Ca)I	Linear mixed, balanced inhibition
HSpI	Hyperbolic specific inhibition
HCaI	Hyperbolic catalytic inhibition
HMx(Sp>Ca)I	Hyperbolic mixed, predominantly specific inhibition
HMx(Sp<Ca)I	Hyperbolic mixed, predominantly catalytic inhibition
HMx(Sp=Ca)I	Hyperbolic mixed, balanced inhibition
HMxD(I/A)	Hyperbolic mixed, dual modification (inhibition → activation)
HCaA	Hyperbolic catalytic activation
HMx(Sp>Ca)A	Hyperbolic mixed, predominantly specific activation
HMxD(A/I)	Hyperbolic mixed, dual modification (activation → inhibition)
HSpA	Hyperbolic specific activation
HMx(Sp<Ca)A	Hyperbolic mixed, predominantly catalytic activation
HMx(Sp=Ca)A	Hyperbolic mixed, balanced activation

The names assigned the mechanisms are logical and are formed using terms suggested in the IUB-Recommendations 1981. The reasons for choosing terms that may not yet be familiar to readers, have been discussed in reference [2, Chapter 3]. Specific (suggested in the Recommendations) in place of competitive and catalytic, in place of uncompetitive (not suggested in the Recommendations) have been used by Cárdenas and Cornish-Bowden [3]. Two new terms had to be created: balanced in place of the deprecated noncompetitive, and dual for mechanisms that display inhibition or activation depending on substrate concentration.